Preliminary Automatics Course
Chapter 12. Integrating unit with inertia
Chapter 12.1 Introduction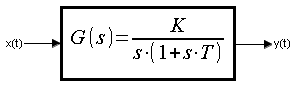
Fig. 12-1
Integrating unit with inertia transfer function
Do you remember an integrating unit? Step type input x(t) caused y(t) increase with steady speed up to infinity. I emphasise. With steady speed. Integrating unit with inertia causes y(t) increase with up to infinity too, but in different from it way. Step type input x(t) causes „scorch” from speed v=0 up to steady v=const. It’s more exactly electrical actuator approximation than an ideal integrating unit.
Chapter 12.1 T=5 sec, step x(t) from the virtual potentiometer, y(t) on the bargraf
Call Desktop/PID/01_podstawowe_człony_dynamiczne/07_calkujacy_z_inercja/01_całkujący_z_inercją_bargraf.zcos
Fig. 12-2
Click „start”.
Fig. 12-3
Move the windows to see 2 digital meters. Virtual potentiometer is set for 0 at the beginning. Set x(t)=+max. Output y(t) is similar to integral unit but the initial y(t) speed=0. Try to stop the y(t). You have to set x(t)=0. The digital meter will be helpful here. When you play it (the y(t) is standing –>no decrease and increase), you see the typical PI or PID attribute. There is nonzero output y(t) value, though input x(t)=0!
Chapter 12.3 k=1 T=3sec x(t) step generator and y(t) oscilloscope.
Call Desktop/PID/01_podstawowe_człony_dynamiczne/07_calkujacy_z_inercja/02_całkujący_z_inercją_skok_oscyloskop.zcos
Fig. 12-4
Integrating unit with inertia = serial connected integrating and inertia units
Click „Start”
Fig. 12-5
Blue yc(t) is after the integrating unit and red y(t) is an output. Do you observe y(t) „scorch” effect at the begining?
I propose the undermentioned objects testing:
FIg. 12-6
We will not call a new block diagram but change the parameters in the existing one. Move right mouse and change k and T parameters.
Fig. 12-7
Your job are the answers for questions.
What does k do?
What does T do?
Chapter 12.4 k=1 T=3sec x(t) single rectangular pulse generator and y(t) oscilloscope.
Wywołaj Pulpit/PID/01_podstawowe_człony_dynamiczne/07_calkujacy_z_inercja/03_całkujący_z_inercją_1_impuls_oscyloskop.zcos
Fig. 12-8
x(t)-single rectangular pulse
Click „Start”
Fig. 12-9
x(t)=input voltage
y(t)= actuator position-angle (valve position for example)
It’s more exactly electrical actuator approximation than ideal integral unit–>yc(t). T is mainly mechanical innertia and a little a electrical motor inertia -cables induction L. It vitiates actuator quality.
Chapter 12.5 k=1 T=3 sec x(t) „+ and -” pulse generator x(t) and y(t) oscilloscope.
Call Desktop/PID/01_podstawowe_człony_dynamiczne/07_calkujacy_z_inercja/04_całkujący_z_inercją_2_impulsy_oscyloskop.zcos
Fig. 12-10
Click „Start”
Fig. 12-11
Blue yc(t)-ideal actuator without inertia (integrating unit)
Red y(t)-real actuator with inertia (integrating with inertia unit). The actuator is „seeking” his valve position. First it find its pos. y(t)=8 and the controller „made a correction” to y(t)=4 then.